 |
cms |
||
ABOUT |
Rick Thomas - Research Interests and Papers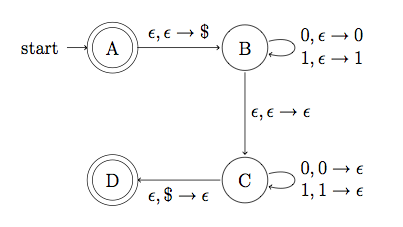 My current research interests. There have been some intriguing interactions in recent years between group theory and theoretical computer science. One area which has proved to be very fruitful in providing interesting and useful results is that of automatic groups. One of my main interests is concerned with extending this theory to semigroups; see [50,53,54,56,57,59,60,67,68,79,83]. The notion of hyperbolic groups has played a fundamental role in computational group theory. I have been interested in extending this theory to monoids with an emphasis on the preservation of efficient algorithms; see [59,71,77]. The word problem for a finitely generated group is one of the must fundamental notions in group theory. One important area is that of investigating what implications the structure of the word problem as a formal language has on the algebraic structure of the corresponding group. There are some related issues here, such as the reduced and irreducible word problems and questions of decidability; see [49,52,55,63,64,66,69,70,76,82,84,86,87,88]. As well as groups, it is possible to consider these issues in the wider context of finitely generated semigroups; several questions that have been completely resolved in the group case remain open in this wider setting. Notwithstanding this, some advances have been made, particularly in the case of the one-counter and context-free languages (see [74,80]). My last main area of current interest is been the classification of which structures are FA-presentable, and there are not many instances where this has been achieved. Amongst the few examples of this to date has been the classification of FA-presentable finitely generated groups in [65] and the generalization to FA-presentable finitely generated cancellative semigroups in [73,75]. Further work on FA-presentable groups and semigroups may be found in [72,78,81].  List of papers.  Copies of any of the papers listed here may be obtained by writing to Professor Richard M. Thomas,Department of Computer Science, University of Leicester, University Road, LEICESTER, LE1 7RH, United Kingdom. or by e-mail to rmt@mcs.le.ac.uk For the older papers, I'm afraid all I have available is the hard copy which I can post to you. For more recent papers, I often have soft copy available as well (normally as a pdf file). Let me know which format you prefer. A list of my research collaborators may be found here.  1. R. M. Thomas, On the centralizers of elements of order 3 in finite groups I, J. Algebra 67 (1980), 163 - 172. 2. R. M. Thomas, On the centralizers of elements of order 3 in finite groups II, J. Algebra 67 (1980), 173 - 184. 3. R. M. Thomas, On the number of generators for certain finite groups, J. Algebra 71 (1981), 576 - 582. 4. R. M. Thomas, On the centralizers of elements of order 3 in finite groups III, J. Algebra 76 (1982), 205 - 210. 5. R. M. Thomas, Some infinite Fibonacci groups, Bull. London Math. Soc. 15 (1983), 384 - 386. 6. R. M. Thomas, On the number of generators for certain finite groups II, J. Algebra 86 (1984), 14 - 22. 7. C. M. Campbell and R. M. Thomas, On infinite groups of Fibonacci type, Proc. Edinburgh Math. Soc. 29 (1986), 225 - 232 8. D. L. Johnson and R. M. Thomas, The Cavicchioli groups are pair-wise non-isomorphic, in E. F. Robertson and C. M. Campbell (eds.), Proceedings of Groups - St Andrews 1985, London Math. Soc. Lecture Notes 121 (Cambridge University Press, 1986), 220 - 222. 9. C. M. Campbell and R. M. Thomas, On (2, n )-groups related to Fibonacci groups, Israel J. Math. 58 (1987), 370 - 380. 10. C. M. Campbell, E. F. Robertson and R. M. Thomas, Fibonacci numbers and groups, in A. F. Horadam, A. N. Philippou and G. E. Bergum (eds.), Applications of Fibonacci Numbers (Kluwer Academic Publishers, 1987), 45 - 59. 11. R. M. Thomas, Cayley graphs and group presentations, Math. Proc. Cambridge Philos. Soc. 103 (1988), 385 - 387.  12. C. M. Campbell, E. F. Robertson and R. M. Thomas, On groups related to Fibonacci groups, in K. N. Cheng and Y. K. Leong (eds.), Group Theory (Walter de Gruyter and Co., 1989), 323 - 331. 13. R. M. Thomas, The Fibonacci groups F(2, 2m ), Bull. London Math. Soc. 21 (1989), 463 - 465. 14. C. M. Campbell, E. F. Robertson and R. M. Thomas, On certain one-relator products of cyclic groups, in A. C. Kim and B. H. Neumann (eds.), Groups Korea - 1988 (Lecture Notes in Mathematics 1398, Springer-Verlag, 1989), 52 - 64. 15. R. M. Thomas, On 2-groups of small rank admitting an automorphism of order p > 3, J.Algebra 125 (1989), 1 - 12. 16. R. M. Thomas, On 2-groups of small rank admitting an automorphism of order 3, J.Algebra 125 (1989), 27 - 35. 17. C. M. Campbell, E. F. Robertson and R. M. Thomas, Finite groups of deficiency zero involving the Lucas numbers, Proc. Edinburgh Math. Soc. 33 (1990), 1 - 10. 18. A. A. Coleman, A. M. Colman and R. M. Thomas, Co-operation without awareness - a multi-person generalization of the minimal social situation, Behavioral Science 35 (1990), 115 - 121. 19. R. M. Thomas, The Fibonacci groups F(4k +2, 4), Comm. Algebra 18 (1990), 3759 - 3763. 20. R. M. Thomas, The Fibonacci groups revisited, in C. M. Campbell and E. F. Robertson (eds.), Proceedings of Groups - St Andrews 1989, Volume 2 (London Math. Soc. Lecture Note Series 160, Cambridge University Press, 1991), 445 - 454. 21. C. M. Campbell, P. M. Heggie, E. F. Robertson and R. M. Thomas, One-relator products of cyclic groups and Fibonacci-like sequences, in G. E. Bergum, A. N. Phillipou and A. F. Horadam (eds.), Applications of Fibonacci Numbers (Kluwer Academic Publishers, 1991), 63 - 68. 22. R. M. Thomas, On a question of Kim concerning certain group presentations, Bull. Korean. Math. Soc. 28 (1991), 219 - 224. 23. R. M. Thomas, On the torsion of certain finitely-presented groups, Comm. Algebra 20 (1992), 469 - 476. 24. R. M. Thomas, Generating sets for finite groups, in W. J. Harvey and C. Maclachlan (eds.), Discrete Groups and Geometry (London Math. Soc. Lecture Notes 173, Cambridge University Press, 1992), 234 - 242. 25. C. M. Campbell, P. M. Heggie, E. F. Robertson and R. M. Thomas, Finite one-relator products of two cyclic groups with the relator of arbitrary length, J. Austral. Math. Soc. 53 (1992), 352 - 368.  26. J. Howie and R. M. Thomas, The groups (2, 3, p; q); asphericity and a conjecture of Coxeter, J. Algebra 154 (1993), 289 - 309. 27. C. M. Campbell, E. F. Robertson and R. M. Thomas, On a class of semigroups with symmetric presentations, Semigroup Forum 46 (1993), 286 - 306. 28. T. Herbst and R. M. Thomas, Group presentations, formal languages and characterizations of one-counter groups, Theoret. Comput. Sci. (Fundamental Study) 112 (1993), 187 - 213. 29. C. M. Campbell, E. F. Robertson and R. M. Thomas, Semigroup presentations and number sequences, in G. E. Bergum, A. N. Phillipou and A. F. Horadam (eds.), Applications of Fibonacci Numbers (Kluwer Academic Publishers, 1993), 77 - 83. 30. C. M. Campbell, P. M. Heggie, E. F. Robertson and R. M. Thomas, Cyclically presented groups embedded in one-relator products of cyclic groups, Proc. Amer. Math. Soc. 118 (1993), 401 - 408. 31. J. Howie and R. M. Thomas, Proving certain groups infinite, in G. A. Niblo and M. A. Roller (eds.), Geometric Group Theory, Volume 1 (London Math. Soc. Lecture Notes 181, Cambridge University Press, 1993), 126 - 131. 32. C. M. Campbell, E. F. Robertson, N. Ruskuc and R. M. Thomas, Fibonacci semigroups, J. Pure Applied Algebra 94 (1994), 49 - 57. 33. C. M. Campbell, E. F. Robertson, N. Ruskuc and R. M. Thomas, Semigroup presentations and minimal ideals, in A. J. Duncan, N. D. Gilbert and J. Howie (eds.), Combinatorial and Geometric Group Theory (London Math. Soc. Lecture Note Series 204, Cambridge University Press, 1995), 29 - 42. 34. C. M. Campbell, E. F. Robertson, N. Ruskuc and R. M. Thomas, Semigroup and group presentations, Bull. London Math. Soc. 27 (1995), 46 - 50. 35. C. M. Campbell, E. F. Robertson, N. Ruskuc and R. M. Thomas, Rewriting a semigroup presentation, Internat. J. Algebra Comput. 5 (1995), 81 - 103. 36. R. M. Thomas, Group presentations where the relators are proper powers, in C. M. Campbell, T. C. Hurley, E. F. Robertson, S. J. Tobin and J. J. Ward (eds.), Groups '93 - Galway / St Andrews, Volume 2 (London Math. Soc. Lecture Notes 212, Cambridge University Press, 1995), 549 - 560.  37. C. M. Campbell, E. F. Robertson, N. Ruskuc and R. M. Thomas, Reidemeister-Schreier type rewriting for semigroups, Semigroup Forum 51 (1995), 47 - 62. 38. J. Howie, V. Metaftsis and R. M. Thomas, Finite generalized triangle groups, Trans. Amer. Math. Soc. 347 (1995), 3613 - 3623. 39. C. M. Campbell, E. F. Robertson, N. Ruskuc and R. M. Thomas, On semigroups defined by Coxeter type presentations, Proc. Royal Soc. Edinburgh 125A (1995), 1063 - 1075. 40. J. Howie, V. Metaftsis and R. M. Thomas, Triangle groups and their generalisations, in A. C. Kim and D. L. Johnson (eds.), Groups - Korea 1994 (Walter de Gruyter, 1995), 135 - 147. 41. E. F. Robertson, R. M. Thomas and C. I. Wotherspoon, A class of inefficient groups with symmetric presentation, in A. C. Kim and D. L. Johnson (eds.), Groups - Korea 1994 (Walter de Gruyter, 1995), 277 - 284. 42. C. M. Campbell, E. F. Robertson, N. Ruskuc, R. M. Thomas and Y. Unlu, On certain one-relator products of semigroups, Comm. Algebra 23 (1995), 5207 - 5219. 43. C. M. Campbell, E. F. Robertson, N. Ruskuc and R. M. Thomas, On subsemigroups of finitely presented semigroups, J. Algebra 180 (1996), 1 - 21. 44. C. M. Campbell, E. F. Robertson, N. Ruskuc and R. M. Thomas, On subsemigroups and ideals in free products of semigroups, Internat. J. Algebra Comput. 6 (1996), 571 - 591. 45. M. Edjvet and R. M. Thomas, The groups (l, m | n, k), J. Pure Applied Algebra 114 (1997), 175 - 208. 46. C. M. Campbell, E. F. Robertson, N. Ruskuc and R. M. Thomas, Presentations for subsemigroups - applications to ideals of semigroups, J. Pure Applied Algebra 124 (1998), 47 - 64. 47. N. Ruskuc and R. M. Thomas, Syntactic and Rees indices of subsemigroups, J. Algebra 205 (1998), 435 - 450. 48. C. M. Campbell, E. F. Robertson, N. Ruskuc and R. M. Thomas, Groups, semigroups and finite presentations, in P. J. Cossey, C. F. Miller III, W. D. Neumann and M. D. Shapiro (eds.), Geometric Group Theory Down Under - Proceedings of a Special Year in Geometric Group Theory, Canberra, Australia. 1996 (de Gruyter, 1999), 55 - 69. 49. I. A. Stewart and R. M. Thomas, Formal languages and the word problem for groups, in C. M. Campbell, E. F. Robertson, N. Ruskuc and G. C. Smith (eds.), Groups St Andrews 1997 in Bath, Volume 2 (London Math. Soc. Lecture Note Series 261, Cambridge University Press, 1999), 689 - 700. 50. M. Edjvet, G. Rosenberger, M. Stille and R. M. Thomas, On certain finite generalized tetrahedron groups, in M. D. Atkinson, N. D. Gilbert, J. Howie, S. A. Linton and E. F. Robertson (eds.), Computational and Geometric Aspects of Modern Algebra (London Math. Soc. Lecture Note Series 275, Cambridge University Press, 2000), 54 - 65.  51. C. M. Campbell, E. F. Robertson, N. Ruskuc and R. M. Thomas, Direct products of automatic semigroups, J. Australian Math. Soc. 69A (2000), 19 - 24. 52. D. W. Parkes and R. M. Thomas, Syntactic monoids and word problems, Arabian J. Science Engineering 25 (2000), 81 - 94. 53. C. M. Campbell, E. F. Robertson, N. Ruskuc and R. M. Thomas, Automatic semigroups, Theoret. Comput. Sci. 365 (2001), 365 - 391. 54. M. Hoffmann and R. M. Thomas, Automaticity and commutative semigroups, Glasgow J. Math. 44 (2002), 167 - 176. 55. D. W. Parkes and R. M. Thomas, Groups with context-free reduced word problem, Comm. Algebra 30 (2002), 3143 - 3156. 56 C. M. Campbell, E. F. Robertson, N. Ruskuc and R. M. Thomas, Automatic completely-simple semigroups, Acta Mathematica Hungarica 95 (2002), 201 - 215. 57. M. Hoffmann, N. Ruskuc and R. M. Thomas, Automatic semigroups with subsemigroups of finite Rees index, Internat. J. Algebra Comput. 12 (2002), 463 - 476. 58. M. Edjvet, J. Howie, G. Rosenberger and R. M. Thomas, Finite generalized tetrahedron groups with a high-power relator, Geometriae Dedicata, 94 (2002), 111 - 139. 59. M. Hoffmann, D. Kuske, F. Otto and R. M. Thomas, Some relatives of automatic and hyperbolic groups, in G. M. S. Gomes, J.-E. Pin and P. V. Silva (eds.), Semigroups, Algorithms, Automata and Languages (World Scientific, 2002), 379 - 406. 60. M. Hoffmann and R. M. Thomas, Notions of automaticity in semigroups, Semigroup Forum, 66 (2003), 337 - 367. 61. G. Rosenberger, M. Scheer and R. M. Thomas, Finite generalized tetrahedron groups with a cubic relator, in C. M. Campbell, E. F. Robertson and G. C. Smith (editors), Groups St Andrews 2001 in Oxford, Volume 2 (London Mathematical Society Lecture Note Series 305, Cambridge University Press, 2003), 455-486. 62. H. Doostie, R. Gholamie and R. M. Thomas, Certain extensions of (l, m | n, k)-groups, Southeast Asian Bulletin of Mathematics 27 (2003), 21-34. 63. D. W. Parkes, V. Yu. Shavrukov and R. M. Thomas, Monoid presentations of groups by finite special string-rewriting systems, RAIRO Theoretical Informatics and Applications 38 (2004), 245-256.  64. S. R. Lakin and R. M. Thomas, Context-sensitive decision problems in groups, in C. S. Calude, E. Calude and M. J. Dinneen (editors), Developments in Language Theory: 8th International Conference, DLT 2004, Auckland, New Zealand (Lecture Notes in Computer Science 3340, Springer-Verlag, 2004), 296-307. 65. G. P. Oliver and R. M. Thomas, Automatic presentations for finitely generated groups, in V. Diekert and B. Durand (editors), 22nd Annual Symposium on Theoretical Aspects of Computer Science (STACS'05), Stuttgart, Germany (Lecture Notes in Computer Science 3404, Springer-Verlag, 2005), 693-704. 66. D. F. Holt, C. E. Rover, S. E. Rees and R. M. Thomas, Groups with a context-free co-word problem, Journal of the London Mathematical Society 71 (2005), 643-657. 67. M. Hoffmann and R. M. Thomas, Biautomatic semigroups, in M. Liskiewicz and R. Reischuk (editors), 15th International Symposium on Fundamentals of Computation Theory (FCT) 2005, Lubeck, Germany (Lecture Notes in Computer Science 3623, Springer-Verlag, 2005), 56-67. 68. M. Hoffmann and R. M. Thomas, A geometric characterization of automatic semigroups, Theoretical Computer Science 369 (2006), 300-313. 69. A. R. Fonseca and R. M. Thomas, Context-free irreducible word problems in groups, in B. Fine, A. M. Gaglione and D. Spellman (editors), Combinatorial Group Theory, Discrete Groups, and Number Theory (Contemporary Mathematics 421, American Mathematical Society, 2006), 125-136. 70. A. R. Fonseca, D. W. Parkes and R. M. Thomas, Irreducible word problems in groups, in C. M. Campbell, M. R. Quick, E. F. Robertson and G. C. Smith (editors), Groups St Andrews 2005, Volume 1 (London Mathematical Society Lecture Note Series 339, Cambridge University Press, 2007), 327-340. 71. M. Hoffmann and R. M. Thomas, Notions of hyperbolicity in monoids, in E. Csuhaj-Varju and Z. Esik (editors), 16th International Symposium on Fundamentals of Computation Theory (FCT) 2007, Budapest, Hungary (Lecture Notes in Computer Science 4639, Springer-Verlag, 2007), 341-352. 72. A. Nies and R. M. Thomas, FA-presentable groups and rings, Journal of Algebra 320 (2008), 569-585. 73. A. J. Cain, G. P. Oliver, N. Ruskuc and R. M. Thomas, Automatic presentations for cancellative semigroups, in C. Martin-Vide, F. Otto and H. Fernau (editors), LATA 2008 (Lecture Notes in Computer Science 5196, Springer-Verlag, 2008), 149-159.  74. D. F. Holt, M. D. Owens and R. M. Thomas, Groups and semigroups with a one-counter word problem,, Journal of the Australian Mathematical Society 85 (2008), 197-209. 75. A. J. Cain, G. P. Oliver, N. Ruskuc and R. M. Thomas, Automatic presentations for semigroups, Information and Computation 207 (2009), 1156-1168. 76. S. R. Lakin and R. M. Thomas, Complexity classes and word problems of groups, Groups, Complexity and Cryptography 1 (2009), 261-273. 77. M. Hoffmann and R. M. Thomas, Notions of hyperbolicity in monoids, Theoretical Computer Science 411 (2009), 799-811. 78. A. J. Cain, G. P. Oliver, N. Ruskuc and R. M. Thomas, Automatic presentations and semigroup constructions, Theory of Computing Systems 47 (2010), 568-592. 79. R. Corran, M. Hoffmann, D. Kuske and R. M. Thomas, Singular Artin monoids of finite type are automatic, in A-H. Dediu, S. Inenaga and C. Martin-Vide (editors), LATA 2011 (Lecture Notes in Computer Science 6638, Springer-Verlag, 2011), 250-261. 80. M. Hoffmann, D. F. Holt, M. D. Owens and R. M. Thomas, Semigroups with a context-free word problem, in H-C. Yen and O. H. Ibarra (editors), Developments in Language Theory: 16th International Conference, DLT 2012, Taipei, Taiwan (Lecture Notes in Computer Science 7410, Springer-Verlag, 2012), 97-108. 81. A. J. Cain, N. Ruskuc and R. M. Thomas, Unary FA-presentable semigroups, International Journal of Algebra and Computation 22 (2012). 82. G. A. Rino Nesin and R. M. Thomas, Groups with a recursively enumerable irreducible word problem, in L. Gasieniec and F. Wolter (editors), 19th International Symposium on Fundamentals of Computation Theory (FCT) 2013, Liverpool, U.K. (Lecture Notes in Computer Science 8070, Springer-Verlag, 2013), 283-292. 83. R. Corran, M. Hoffmann, D. Kuske and R. M. Thomas, On the automaticity of singular Artin monoids of finite type, International Journal of Computer Mathematics 90 (2013), 1197-1222. 84. S. A. M. Jones and R. M. Thomas, Formal languages, word problems of groups and decidability, in P. A. Abdulla and I. Potapov (editors), Reachability Problems - 7th International Workshop, RP 2013, Uppsala, Sweden, 2013 (Lecture Notes in Computer Science 8169, Springer-Verlag, 2013), 146-158. 85. G. A. Rino Nesin and R. M. Thomas, Groups whose word problem is a Petri net language, in J. Shallit and A. Okhotin (editors), 17th International Workshop, DCFS 2015, Waterloo, Canada (Lecture Notes in Computer Science 9118, Springer-Verlag, 2015), 243–255. 86. S. A. M. Jones and R. M. Thomas, Formal languages and group theory, in C. M. Campbell, M. R. Quick, E. F. Robertson and C. M. Roney-Dougal (editors), Proceedings of Groups St Andrews 2013 (London Mathematical Society Lecture Note Series 422, Cambridge University Press, 2015), 306–323. 87. S. A. M. Jones and R. M. Thomas, Characterizing word problems of groups, in K. G. Larsen, I. Potapov and J. Srba (editors), Reachability Problems - 10th International Workshop, RP 2016, Aalborg, Denmark, 2016 (Lecture Notes in Computer Science 9899, Springer-Verlag, 2016), 104-118. 88. S. A. M. Jones and R. M. Thomas, Word problems of groups: formal languages, characterizations and decidability, Theoretical Computer Science 750 (2018), 2-23. Whilst I answer to "Rick" my official name is "Richard" and that is what I put on my publications; hence the "Richard M Thomas" you will see on my papers. I don't normally use my middle name but, if you want to know what it is, the phrase "nomadic maths horror" is a (to my mind rather appropriate) anagram of my full name. To avoid any possible confusion here, I should point out that my middle name is not "moron"! |
Author:
Rick Thomas (rmt@mcs.le.ac.uk). |
|